British language expert shares 7 American accents that are particularly tricky
Some of these are even challenging for other Americans to understand.
Have you ever heard these 7 unique American accents?
When you think about "American English," what comes to mind? The way we us a hard "r" compared to British English? The way we pronounce "aluminum" and "herbs"? How we say "cookies" instead of "biscuits" and "fries" instead of "chips"?
What about our different accents? While we often marvel about how many accents there are in the U.K. for its size, the U.S has a lot more diversity of accents than people might think. Most of us group American accents into large regional groupings like Northeast, Southern, Midwest, etc., and the people from each of those regions know that there are distinct accents within them (like Boston vs. New York, Tennessee vs. Mississippi). But there are even more hyper-localized accents and dialects that many of us are not exposed to, and some of them are hard even for other Americans to understand.
Language expert Olly Richards shared seven of these accents that are difficult to emulate and explained how they came to be. It's a fascinating celebration of the diversity that many of us aren't aware exists within our language.
- YouTube www.youtube.com
1. The YAT accent of New Orleans, Louisiana
Influenced by West African, German, Italian, Irish, and French immigrants who settled in Louisiana, the YAT accent is named for the way people say "Where you at?" in New Orleans. Words and phrases from this accent include "dawlin" (darling) "Mawmaw" (grandma) "prolly" (probably), "pass a good time" (have a good time), "Who dat?" (Who's that?), and "for noon" (at noon).
The YAT accent is distinct from a southern drawl or from the local Cajun English—it's more akin to a thick New York accent, which Richards says is due to the exact same immigrant groups settling there.
2. The High Tider accent of Outer Banks, North Carolina
Richards calls this an "endangered accent," as it appears to be dying out. It stems from immigration from the south of England and Ireland, but also from pirates of varying backgrounds who used the islands off the coast of North Carolina. The High Tider dialect developed in almost complete isolation for over 250 years, and is a form of archaic English that includes elements found in parts of Canadian English as well. A few words from this dialect include "quamish" (sick or nauseated), "buck" (male friend), and "dingbatter" (outsider).

3. The Miami English accent of Miami, Florida
Sitting at the tip of Florida, Miami has been heavily influenced by five decades of immigration from Cuba and other parts of the Caribbean as well as Central America. As a result, the English has many elements of Spanish pronunciations and rhythm. English has 20 vowel sounds, but Spanish has only five, so the Miami English accent reflects those Spanish vowel sounds.
4. The Southerner accent of the American South
Okay, this one is a bit fudgey because, as Richards points out, there are actually seven distinct southern accents. But there are some elements that Southerner accents share, one being how they speak more slowly than most of the rest of the country. Southern accent are known for their melodic nature and long, drawn-out vowels. Richards explains that wealthy British traders living in this part of the U.S. in the mid-1700s started dropping their "r" sound as a way to distinguish themselves from the lower classes. We can still hear that characteristic in southern accents today.
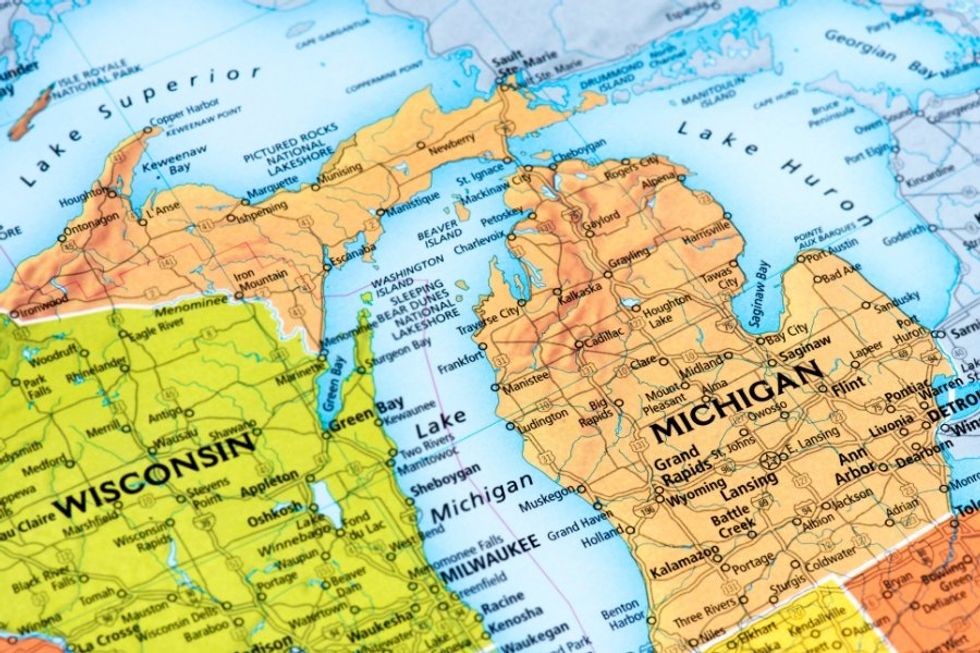
5. The Yooper accent of the Upper Midwest
This accent has its roots in German, Scandinavian, Cornish, French Canadian, and particularly Finnish, and is perhaps most recognized by its affinity for its use of the German-Scandinavian "ja" instead of "yes" and for ending sentences with "eh." Another feature is saying "dem/dere/dat" instead of them/there/that. The term "Yooper" comes from "U.P." or "Upper Peninsula," referring to the northern part of Michigan, but variations of this accent can be found along various parts of the Midwest near the Canadian border.
6. The Mainer accent of Maine
This accent is marked by the dropping of "r" sounds, but also adding an "r" where there shouldn't be one (similarly to the way British folks treat "r"). There also tends to be a slight gruffness in the throat when they speak. The accent is influenced by 17th century English and early French settlers, along with a bit of Scots-Irish. Words and phrases like "from away" (not from Maine), "drownded" (drown), "brung" (bring), and "chuppta" (What are you up to?) are common, and though its association with the working class caused it to dwindle, Richards says the Mainer dialect is having a bit of a renaissance.

7. The Gullah accent of the Carolinas, Georgia, and Florida
This unique accent developed in the southern rice fields during the Atlantic slave trade. The enslaved peoples learned English, but it was heavily influenced by the various African languages they spoke, as well as the lower-class English and Irish servants they interacted with. Today, it's mostly African-Americans living along the Atlantic seaboard of North and South Carolina, Georgia, and Florida who use the Gullah dialect. The word "Kumbaya" comes from Gullah, which Richards describes as more than an accent or dialect—it's actually an American creole (a language that evolved from two other languages coming together).
It's easy to imagine the "standard" American English accent seen most often in Hollywood films and TV shows, which most closely aligns with the Western U.S., but in reality, American English is a mishmash of accents and dialects that are more diverse than many of us even know. What a delightful celebration of the English language in all of its colorful manifestations.

 A man handing a woman his business card at an event.via
A man handing a woman his business card at an event.via  A man and woman sharing a drink at a bar.via
A man and woman sharing a drink at a bar.via  Rob Lowe in a scene from Parks And Recreation
Rob Lowe in a scene from Parks And Recreation  A can of spams is also a spam.
A can of spams is also a spam.  A man making a sales presentation.via
A man making a sales presentation.via A man with his hands open making a speech.via
A man with his hands open making a speech.via A woman reading a book about house plants.via
A woman reading a book about house plants.via  A woman listening to music in bedvia
A woman listening to music in bedvia  Imperial eras of time. Canva Photos.
Imperial eras of time. Canva Photos. sailor moon walking GIF
sailor moon walking GIF